ISEE Upper Level
Mathematics Practice Test 1
- 47 questions
- Total time for this section: 40 Minutes
- Calculators are not allowed at the test.
|
| 1- |
Which of the following graphs represents the compound inequality 3 ≤ 2 x − 3 < 13? |
| (A) |
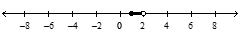 |
| (B) |
 |
| (C) |
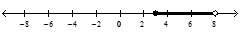 |
| (D) |
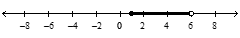 |
| 2- |
Which graph shows a non-proportional linear relationship between x and y? |
| (A) |
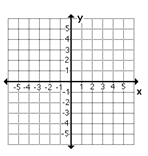 |
| (B) |
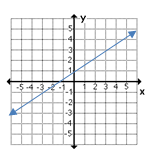 |
| (C) |
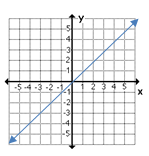 |
| (D) |
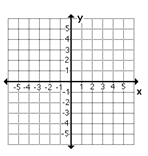 |
| 3- |
A girl 162 cm tall, stands 270 cm from a lamp post at night. Her shadow from the light is 100 cm long. How high is the lamp post?
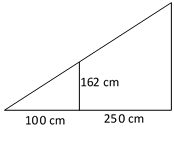 |
| (A) |
800 cm |
| (B) |
650 cm |
| (C) |
576 cm |
| (D) |
317 cm |
| 4- |
Find all values of x for which 2 x2 + 15 x + 7=0 |
| (A) |
− 7,− 13 |
| (B) |
8,12 |
| (C) |
− 7,− 12 |
| (D) |
− 35,− 12 |
| 5- |
Solve.
|1 \ – \ (12 \ ÷ \ | 1 \ - \ 5 |) | =? |
| (A) |
2 |
| (B) |
- \ 2 |
| (C) |
- \ 5 |
| (D) |
5 |
| 6- |
The ratio of boys to girls in a school is 7:4. If there are 550 students in a school, how many boys are in the school? |
| (A) |
350 |
| (B) |
250 |
| (C) |
200 |
| (D) |
400 |
| 7- |
The rectangle on the coordinate grid is translated 5 units down and 4 units to the left.
Which of the following describes this transformation?
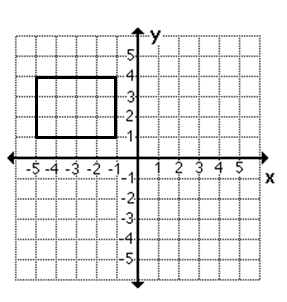 |
| (A) |
(x,y) ⇒ (x \ - \ 4, y \ + \ 5) |
| (B) |
(x,y) ⇒ (x \ - \ 4, y \ - \ 5) |
| (C) |
(x,y) ⇒ (x \ + \ 4, y \ + \ 5) |
| (D) |
(x,y) ⇒ (x \ + \ 4, y \ - \ 5) |
| 8- |
How is this number written in scientific notation?
0.0000007685 |
| (A) |
7.685 \ × \ 10^{–7} |
| (B) |
76.85 \ × \ 10^{–9} |
| (C) |
7.685 \ × \ 10^{4} |
| (D) |
0.0007685 \ × \ 10^{- \ 8} |
| 9- |
(x \ - \ 9) \ (x \ + \ 3) = |
| (A) |
x^2 \ + \ 8 \ x \ - \ 22 |
| (B) |
2 \ x \ + \ 32 \ x \ - \ 27 |
| (C) |
- \ x^2 \ - \ 6 \ x \ + \ 27 |
| (D) |
x^2 \ - \ 6 \ x \ - \ 27 |
| 10- |
Which value of x makes the following inequality true?
\frac{2}{25} \ ≤ \ x \ < \ 25\% |
| (A) |
0.27 |
| (B) |
\frac{7}{48} |
| (C) |
\sqrt{0.169} |
| (D) |
0.2981 |
| 11- |
Use the diagram below to answer the question.
Given the lengths of the base and diagonal of the rectangle below, what is the length of height h, in terms of s?
 |
| (A) |
2 \ s \ \sqrt{5} |
| (B) |
2 \ s^2 |
| (C) |
s \ \sqrt{15} |
| (D) |
4 \ s |
| 12- |
If the area of trapezoid is 168 cm^2, what is the perimeter of the trapezoid?
 |
| (A) |
40 \ + \ 4 \ \sqrt{ 10 } cm |
| (B) |
4 \ \sqrt{ 13 } cm |
| (C) |
10 \ \sqrt{ 3} cm |
| (D) |
10 \ + \ 4 \ \sqrt{ 10} cm |
| 13- |
Find the area of a rectangle with a length of 145 feet and a width of 68 feet. |
| (A) |
10,340 sq. ft. |
| (B) |
12,602 sq. ft. |
| (C) |
9,860 sq. ft. |
| (D) |
8,540 sq. ft. |
| 14- |
Emily and Daniel have taken the same number of photos on their school trip. Emily has taken 3 times as many as photos as Claire and Daniel has taken 12 more photos than Claire. How many photos has Claire taken? |
| (A) |
6 |
| (B) |
8 |
| (C) |
4 |
| (D) |
10 |
| 15- |
93 \ ÷ \ \frac{1}{5} =? |
| (A) |
512 |
| (B) |
610 |
| (C) |
46.5 |
| (D) |
465 |
| 16- |
3 \ – \ 12 \ ÷ \ (5^2 \ ÷ \ 5) = ___ |
| (A) |
- \ 1 |
| (B) |
4 |
| (C) |
8 |
| (D) |
- \ 3 |
| 17- |
Emily lives 4 \ \frac{1}{5} miles from where she works. When traveling to work, she walks to a bus stop \frac{1}{2} of the way to catch a bus. How many miles away from her house is the bus stop? |
| (A) |
3 \ \frac{1}{5} Miles |
| (B) |
2 \ \frac{1}{10} Miles |
| (C) |
2 \ \frac{1}{5} Miles |
| (D) |
4 \ \frac{1}{10} Miles |
| 18- |
\frac{8}{32} is equals to: |
| (A) |
0.25 |
| (B) |
2.15 |
| (C) |
0.025 |
| (D) |
1.025 |
| 19- |
Use the chart below to answer the question.
There are also purple marbles in the bag. Which of the following can NOT be the probability of randomly selecting a purple marble from the bag?
 |
| (A) |
\frac{1}{10} |
| (B) |
\frac{1}{4} |
| (C) |
\frac{2}{5} |
| (D) |
\frac{7}{15} |
| 20- |
If (3.2 \ + \ 3.6 \ + \ 3.8) \ x = x, then what is the value of x? |
| (A) |
10.6 |
| (B) |
1 |
| (C) |
0 |
| (D) |
\frac{1}{10.6} |
| 21- |
Two dice are thrown simultaneously, what is the probability of getting a sum of 6 or 9? |
| (A) |
\frac{1}{3} |
| (B) |
\frac{1}{4} |
| (C) |
\frac{1}{25} |
| (D) |
\frac{1}{12} |
| 22- |
If a vehicle is driven 25 miles on Monday, 40 miles on Tuesday, and 22 miles on Wednesday, what is the average number of miles driven each day? |
| (A) |
32 Miles |
| (B) |
31 Miles |
| (C) |
30 Miles |
| (D) |
29 Miles |
| 23- |
In the following figure, AB is the diameter of the circle. What is the circumference of the circle?
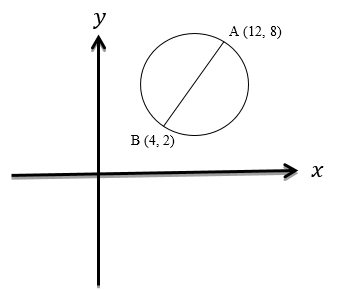 |
| (A) |
5 \ \pi |
| (B) |
20 \ \pi |
| (C) |
10 \ \pi |
| (D) |
15 \ \pi |
| 24- |
48.32 \ ÷ \ 0.05 =? |
| (A) |
900.45 |
| (B) |
850.18 |
| (C) |
966.4 |
| (D) |
950.52 |
| 25- |
Solve for 4 \ x^2 \ + \ 8 = 24 |
| (A) |
± \ 4 |
| (B) |
± \ 8 |
| (C) |
± \ 16 |
| (D) |
± \ 2 |
| 26- |
With an 25\% discount, Ella was able to save $28.32 on a dress. What was the original price of the dress? |
| (A) |
$113.28 |
| (B) |
$120.10 |
| (C) |
$112.94 |
| (D) |
$121.32 |
| 27- |
Use the following table to answer question below.
This table shows the data Daniel collects while watching birds for one week. How many raptors did Daniel see on Monday?
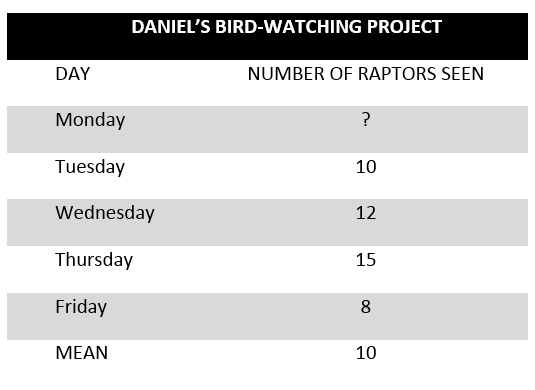 |
| (A) |
5 |
| (B) |
10 |
| (C) |
15 |
| (D) |
20 |
| 28- |
A circle has a diameter of 18 inches. What is its approximate area? |
| (A) |
254.34 in^2 |
| (B) |
269.22 in^2 |
| (C) |
310.93 in^2 |
| (D) |
300.45 in^2 |
| 29- |
The sum of 6 numbers is greater than 240 and less than 380. Which of the following could be the average (arithmetic mean) of the numbers? |
| (A) |
35 |
| (B) |
75 |
| (C) |
55 |
| (D) |
25 |
$24.99 $16.99
32% Off*
The Ultimate Step by Step Guide to Preparing for the ISEE Upper Level Math Test
|
| 30- |
Simplify \frac{\frac{1}{3} \ - \ \frac{x \ + \ 4}{2}}{\frac{x^2}{2} \ - \ \frac{3}{4}} |
| (A) |
\frac{ - \ 6 \ x \ - \ 20}{6 \ x^2 \ - \ 9} |
| (B) |
\frac{ - \ 3 \ x \ + \ 10}{6 \ x^2 \ + \ 6} |
| (C) |
\frac{12 \ x \ + \ 10}{ \ x^2 \ - \ 9} |
| (D) |
\frac{8 \ x \ - \ 12}{4 \ x^2 \ + \ 9} |
| 31- |
The base of a right triangle is 4 foot, and the interior angles are 45-45-90. What is its area? |
| (A) |
16 square feet |
| (B) |
8 square feet |
| (C) |
4 square feet |
| (D) |
4.5 square feet |
| 32- |
If 5 garbage trucks can collect the trash of 25 homes in a day. How many trucks are needed to collect in 200 houses? |
| (A) |
30 |
| (B) |
40 |
| (C) |
25 |
| (D) |
45 |
| 33- |
Which equation represents the statement twice the difference between 4 times H and 2 gives 25. |
| (A) |
3 \ (2 \ H \ - \ 4) = 25 |
| (B) |
\frac{1}{2} \ (4 \ H \ + \ 2) = 25 |
| (C) |
2 \ (4 \ H \ - \ 2) = 25 |
| (D) |
4 \ (2 \ H \ - \ 25) = 2 |
| 34- |
A floppy disk shows 840,128 bytes free and 698,589 bytes used. If you delete a file of size 552,129 bytes and create a new file of size 439,566 bytes, how many free bytes will the floppy disk have? |
| (A) |
892,691 |
| (B) |
1032,623 |
| (C) |
983,123 |
| (D) |
952,691 |
| 35- |
If 4 \ + \ x^{\frac{1}{2)}}=20, then what is the value of 15 \ × \ x? |
| (A) |
3,340 |
| (B) |
3,840 |
| (C) |
2,925 |
| (D) |
3,125 |
| 36- |
The width of a box is one third of its length. The height of the box is one third of its width. If the length of the box is 36 cm, what is the volume of the box? |
| (A) |
1,728 cm^3 |
| (B) |
1,548 cm^3 |
| (C) |
1,317 cm^3 |
| (D) |
1,250 cm^3 |
| 37- |
Triangle ABC is graphed on a coordinate grid with vertices at A (- \ 3, - \ 2), B (- \ 1, 4) and C (7, 9).
Triangle ABC is reflected over x axes to create triangle A’B’C’.
Which order pair represents the coordinate of C’? |
| (A) |
(7, 9) |
| (B) |
(7, - \ 9) |
| (C) |
(- \ 7, - \ 9) |
| (D) |
(- \ 7, 9) |
| 38- |
7 days 21 hours 21 minutes – \ 4 days 14 hours 14 minutes =? |
| (A) |
3 days 7 hours 7 minutes |
| (B) |
3 days 7 hours 14 minutes |
| (C) |
2 days 6 hours 21 minutes |
| (D) |
2 days 7 hours 19 minutes |
| 39- |
A square measures 6 inches on one side. By how much will the area be decreased if its length is increased by 5 inches and its width decreased by 3 inches. |
| (A) |
2 sq decreased |
| (B) |
1 sq decreased |
| (C) |
4 sq decreased |
| (D) |
3 sq decreased |
| 40- |
A circle is inscribed in a square, as shown below.
The area of the circle is 24 \ π cm^2. What is the area of the square?
 |
| (A) |
100 cm^2 |
| (B) |
80 cm^2 |
| (C) |
50 cm^2 |
| (D) |
150 cm^2 |
| 41- |
6 \ x^2 \ y^3 \ + \ 2 \ x^3 \ y^5 \ – \ (8 \ x^2 \ y^3 \ – \ 3 \ x^3 \ y^5) = ___ |
| (A) |
2 \ x^2 \ y^3 \ - \ 5 \ x^3 \ y^5 |
| (B) |
3 \ x^2 \ y^3 \ - \ 7 \ x^3 \ y^5 |
| (C) |
- \ 2 \ x^2 \ y^3 \ + \ 5 \ x^3 \ y^5 |
| (D) |
- \ 5 \ x^2 \ y^3 \ - \ 2 \ x^3 \ y^5 |
| 42- |
Increased by 45\%, the numbers 91 becomes: |
| (A) |
122.31 |
| (B) |
110.87 |
| (C) |
131.95 |
| (D) |
140.12 |
| 43- |
How many 3 \ × \ 3 squares can fit inside a rectangle with a height of 35 and width of 18? |
| (A) |
70 |
| (B) |
73 |
| (C) |
62 |
| (D) |
52 |
| 44- |
The radius of circle A is three times the radius of circle B. If the circumference of circle A is 12 \ π, what is the area of circle B? |
| (A) |
8 \ 𝜋 |
| (B) |
4 \ 𝜋 |
| (C) |
12 \ 𝜋 |
| (D) |
10 \ 𝜋 |
| 45- |
Which set of ordered pairs represents y as a function of x? |
| (A) |
\left\{(3, - \ 2), (3, 7), (9, - \ 8), (4, - \ 7)\right\} |
| (B) |
\left\{(4, 2), (3, - \ 9), (5, 8), (4, 7)\right\} |
| (C) |
\left\{(9, 12), (5, 7), (6, 11), (5, 18)\right\} |
| (D) |
\left\{(6, 1), (3, 1), (0, 5), (4, 5)\right\} |
| 46- |
David makes a weekly salary of $350 plus 10\% commission on his sales. What will his income be for a week in which he makes sales totaling $1,500? |
| (A) |
$500 |
| (B) |
$540 |
| (C) |
$480 |
| (D) |
$620 |
| 47- |
If a box contains red and blue balls in ratio of 4 : 5 red to blue, how many red balls are there if 50 blue balls are in the box? |
| (A) |
60 |
| (B) |
40 |
| (C) |
50 |
| (D) |
70 |