|
ACCUPLACER Mathematics Practice Test 4
(Non–Calculator) 2 Sections – 40 questions
Total time for two sections: No Time Limit You may not use a calculator on this section.
|
| Arithmetic and Elementary Algebra |
| 1- |
Last Friday Jacob had $45.12 . Over the weekend he received some money for cleaning the attic. He now has $62 . How much money did he receive? |
| (A) |
$16.68 |
| (B) |
$16.78 |
| (C) |
$16.88 |
| (D) |
$16.98 |
| 2- |
x2 − 8 x + 15 = ? |
| (A) |
(x \ – \ 3) \ (x \ - \ 5) |
| (B) |
(x \ + \ 3) \ (x \ - \ 5) |
| (C) |
(x \ + \ 3) \ (x \ + \ 5) |
| (D) |
(x \ - \ 3) \ (x \ + \ 5) |
| 3- |
What is 6254.74164 rounded to the nearest tenth? |
| (A) |
6254.7 |
| (B) |
6254.8 |
| (C) |
6254.9 |
| (D) |
6254.0 |
| 4- |
In the following diagram, what is the value of x in the following triangle?
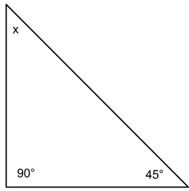 |
| (A) |
40^\circ |
| (B) |
46^\circ |
| (C) |
60^\circ |
| (D) |
45^\circ |
| 5- |
45 is what percent of 120 ? |
| (A) |
37\% |
| (B) |
37.5\% |
| (C) |
38.5\% |
| (D) |
38\% |
| 6- |
Which of the following equations has a graph that is a straight line? |
| (A) |
3 \ y \ + \ 6 = 4 \ x |
| (B) |
y \ = \ 3 \ x^2 \ + \ 9 |
| (C) |
3 \ y^2 \ + \ 6 = x |
| (D) |
3 \ y \ + \ 6 = x^2 |
| 7- |
Find all values for which 2 \ x^2 \ - \ 5 \ x \ - \ 3 \ = \ 0 |
| (A) |
- \ 12 , \frac{1}{2} |
| (B) |
\frac{1}{2} , 12 |
| (C) |
- \ \frac{1}{2} , 3 |
| (D) |
- \ \frac{3}{2} , 12 |
| 8- |
What is the distance between the points (2, 4) and (- \ 4, - \ 4) ? |
| (A) |
100 |
| (B) |
10 |
| (C) |
5 |
| (D) |
7 |
| 9- |
Simplify \frac{\frac{4}{3} \ - \ \frac{2 \ x \ - \ 3}{9}}{\frac{x^2}{3} \ - \ \frac{7}{3}} |
| (A) |
\frac{- \ 2 \ x \ - \ 15}{3 \ x^2 \ - \ 21} |
| (B) |
\frac{- \ 2 \ x \ - \ 15}{3 \ x^2 \ +\ 21} |
| (C) |
\frac{- \ 2 \ x \ + \ 15}{3 \ x^2 \ + \ 21} |
| (D) |
\frac{- \ 2 \ x \ + \ 15}{3 \ x^2 \ - \ 21} |
| 10- |
A man owed $ 3254 on his car. After making 42 payment of $ 65 each, how much did he have left to pay? |
| (A) |
$ 234 |
| (B) |
$ 236 |
| (C) |
$ 524 |
| (D) |
$ 253 |
| 11- |
Write the \frac{7}{120} as a decimal. |
| (A) |
0.059 |
| (B) |
0.058 |
| (C) |
0.60 |
| (D) |
0.62 |
| 12- |
\sqrt{75} is between which two whole numbers? |
| (A) |
7 and 8 |
| (B) |
8 and 9 |
| (C) |
6 and 7 |
| (D) |
5 and 6 |
| 13- |
Which of the following is one solution of this equation?
3 \ x^2 \ + \ 10 \ x \ - \ 2 \ = \ 0 |
| (A) |
\frac{- \ 5 \ ± \ \sqrt{31}}{3} |
| (B) |
\frac{- \ 5 \ - \ \sqrt{31}}{3} , 0.12 |
| (C) |
\frac{- \ 5 \ + \ \sqrt{31}}{3} , 0.358 |
| (D) |
\frac{- \ 5 \ + \ \sqrt{31}}{3} , 0.438 |
| 14- |
(x \ + \ 7 ) \ (x^{2} \ - \ 4 \ x \ + \ 3) = ? |
| (A) |
x^3 \ - \ 3 \ x^2 \ – \ 25 \ x \ + \ 21 |
| (B) |
x^3 \ - \ 3 \ x^2 \ + \ 25 \ x \ + \ 21 |
| (C) |
x^3 \ - \ 3 \ x^2 \ + \ 25 \ x \ - \ 21 |
| (D) |
x^3 \ + \ 3 \ x^2 \ – \ 25 \ x \ + \ 21 |
| 15- |
(x^{5})^{\frac{2}{7}} |
| (A) |
x^{ \frac{11}{7}} |
| (B) |
x^{ \frac{10}{7}} |
| (C) |
x^{ \frac{9}{7}} |
| (D) |
x^{ \frac{8}{7}} |
| 16- |
How many 6 \ × \ 6 squares can fit inside a rectangle with a height of 42 and width of 18? |
| (A) |
21 |
| (B) |
16 |
| (C) |
24 |
| (D) |
36 |
| 17- |
If a vehicle is driven 42 miles on Monday, 47 miles on Tuesday, and 31 miles on Wednesday, what is the average number of miles driven each day? |
| (A) |
42 miles |
| (B) |
45 miles |
| (C) |
46 miles |
| (D) |
40 miles |
| 18- |
Alex’s average (arithmetic mean) on two mathematics tests is 10 . What should Liam’s score be on the next test to have an overall of 12 for all the tests? |
| (A) |
12 |
| (B) |
18 |
| (C) |
16 |
| (D) |
10 |
| 19- |
If 6\ - \ 3 \ x \ ≤ \ 18 , what is the value of x \geq ? |
| (A) |
4 |
| (B) |
3 |
| (C) |
-4 |
| (D) |
-3 |
| 20- |
9^{5 } \ × \ 9^{ - \ 8} \ = ? |
| (A) |
9^{ 3} |
| (B) |
9^{ - \ 3} |
| (C) |
9^{ 14} |
| (D) |
9^{ 10} |
$24.99 $13.99
44% Off*
The Ultimate Step by Step Guide to Preparing for the Accuplacer Math Test
|
| College–Level Mathematics |
| 21- |
cos 2 \ \theta = ? |
| (A) |
1\ + \ 2 \ sin^2\ \theta |
| (B) |
1\ - \ 2 \ sin^2\ \theta |
| (C) |
- \ 2 \ sin^2\ \theta |
| (D) |
2 \ sin^2\ \theta |
| 22- |
If \theta is an acute angle and sin \theta =\frac{4}{5} , cos \theta = ? |
| (A) |
\frac{4}{5} |
| (B) |
\frac{3}{5} |
| (C) |
\frac{2}{5} |
| (D) |
\frac{1}{5} |
| 23- |
If the center of a circle is at the point (4, - \ 1) and its circumference equals to 4 \ π , what is the standard form equation of the circle? |
| (A) |
(x \ +\ 4)^2 \ + \ (y \ + \ 1)^2 = 2 |
| (B) |
(x \ - \ 4)^2 \ + \ (y \ + \ 1)^2 \ = \ 4 |
| (C) |
(x \ - \ 4)^2 \ + \ (y \ + \ 1)^2 \ = \ 2 |
| (D) |
(x \ - \ 4)^2 \ + \ (y \ - \ 1)^2 \ = \ 4 |
| 24- |
What is the solution of the following system of equations?
\begin{cases} 4 \ x \ + \ y = 8 \\ - \ 8 \ x \ - \ 4 \ y = 16\end{cases} |
| (A) |
(6, 16) |
| (B) |
(- \ 6, 16) |
| (C) |
(6, - \ 16) |
| (D) |
(6, 16) |
| 25- |
What is the center and radius of a circle with the following equation?
(x \ – \ 6)^2 \ + \ (y \ + \ 4)^2 \ = \ 5 |
| (A) |
(6, 4), \ \sqrt{5} |
| (B) |
(6 , - \ 4), \ \sqrt{5} |
| (C) |
(- \ 6 , - \ 4), \ \sqrt{5} |
| (D) |
(- \ 6 , 4), \ \sqrt{5} |
| 26- |
If sin A =\ \frac{2}{5} in a right triangle and the angle A is an acute angle, then what is cos A ? |
| (A) |
\frac{\sqrt{22}}{5} |
| (B) |
\frac{\sqrt{20}}{5} |
| (C) |
\frac{\sqrt{21}}{5} |
| (D) |
\frac{\sqrt{3}}{5} |
| 27- |
If \log_{3}{x \ = \ 6} , then x \ = ? |
| (A) |
729 |
| (B) |
243 |
| (C) |
719 |
| (D) |
216 |
| 28- |
Simplify: \frac{2 \sqrt{12}}{9 \sqrt{48}} |
| (A) |
\frac{2}{9} |
| (B) |
\frac{4}{9} |
| (C) |
\frac{1}{9} |
| (D) |
\frac{5}{9} |
| 29- |
What’s the reciprocal of \frac{25}{x^3} ? |
| (A) |
\frac{x^3}{25 } |
| (B) |
\frac{25}{x^3 } |
| (C) |
\frac{5}{x } |
| (D) |
\frac{5}{x ^3 } |
| 30- |
If \log_{4}{x \ = \ 3} , then x \ = ? |
| (A) |
81 |
| (B) |
16 |
| (C) |
64 |
| (D) |
36 |
| 31- |
What is sin 60^\circ ? |
| (A) |
\frac{\sqrt{3}}{2} |
| (B) |
\sqrt{3} |
| (C) |
- \ \sqrt{3} |
| (D) |
- \ \frac{\sqrt{3}}{2} |
| 32- |
Find the inverse function for ln (4 \ x \ - \ 3) |
| (A) |
\frac{1}{4} (e^{\ x} \ - \ 3) |
| (B) |
\frac{1}{2} (e^{\ x} \ + \ 3) |
| (C) |
\frac{1}{4} (e^{\ x} \ + \ 3) |
| (D) |
\frac{1}{2} (e^{\ x} \ - \ 3) |
| 33- |
Solve.
| \ 15 \ – \ (18 \ ÷ \ | \ 3 \ + \ 6 \ |)| = ? |
| (A) |
- \ 13 |
| (B) |
- \ 12 |
| (C) |
12 |
| (D) |
13 |
| 34- |
Simplify ( – \ 7 \ + \ 2 \ i) \ (9 \ + \ 4 \ i) . |
| (A) |
- \ 10 \ i \ - \ 71 |
| (B) |
- \ 10 \ i \ + \ 71 |
| (C) |
10 \ i \ + \ 71 |
| (D) |
10 \ i \ - \ 71 |
| 35- |
If f(x) \ = \ x \ – \frac{4}{5} and f ^{ \ – \ 1} is the inverse of f(x) , what is the value of f^{ \ - \ 1}(2) |
| (A) |
\frac{7}{5} |
| (B) |
\frac{16}{5} |
| (C) |
\frac{6}{5} |
| (D) |
\frac{14}{5} |
| 36- |
Find tan \frac{4 \ π}{3} |
| (A) |
- \ \sqrt{3} |
| (B) |
\sqrt{3} |
| (C) |
2 \ \sqrt{3} |
| (D) |
- \ 2 \ \sqrt{3} |
| |
| 37- |
Solve the equation: \log_{5}{(x \ - \ 4)} \ – \ \log_{5}{(x \ + \ 3)} \ = \ 1 |
| (A) |
\frac{9}{4} |
| (B) |
\frac{18}{4} |
| (C) |
\frac{14}{4} |
| (D) |
-\frac{19}{4} |
| 38- |
If f(x) \ = 2 \ x \ + \ 6 and g(x) \ = x^2 \ + \ 3 \ x , then find (\frac{f}{g}) (x) |
| (A) |
\frac{2 \ x \ - \ 6}{x^2 \ +\ 3 \ x} |
| (B) |
\frac{2 \ x \ + \ 6}{x^2 \ +\ 3 \ x} |
| (C) |
\frac{2 \ x \ + \ 6}{x^2 \ - \ 3 \ x} |
| (D) |
\frac{2 \ x \ - \ 6}{x^2 \ - \ 3 \ x} |
| 39- |
The slop of a line with the equation y \ = \ 6 \ x \ + \ 12 is … |
| (A) |
4 |
| (B) |
\frac{5}{3} |
| (C) |
\frac{5}{6} |
| (D) |
6 |
| 40- |
Solve e^{(7 \ x \ + \ 1 )} \ = \ 8 |
| (A) |
\frac{ln(8) \ +\ 1}{7} |
| (B) |
\frac{ln(8) \ +\ 1}{5} |
| (C) |
\frac{ln(8) \ -\ 1}{5} |
| (D) |
\frac{ln(8) \ -\ 1}{7} |