|
ISEE Middle Level
Practice Test 3
Quantitative Reasoning
- 37 questions
- Total time for this section: 35 Minutes
- Calculators are not allowed at the test.
|
| 1- |
Solve. − 45 × 0.25 |
| (A) |
− 95 |
| (B) |
− 75 |
| (C) |
45 |
| (D) |
− 2 |
| 2- |
Jim purchased a table for 10% off and saved $25. What was the original price of the table? |
| (A) |
$250 |
| (B) |
$240 |
| (C) |
$220 |
| (D) |
$230 |
| 3- |
The area of a circle is 49 \ π in^2. What is the circumference of the circle? |
| (A) |
16 \ π |
| (B) |
12 \ π |
| (C) |
14 \ π |
| (D) |
18 \ π |
| 4- |
What is the value of x in the following equation? 9^x=729 |
| (A) |
2 |
| (B) |
3 |
| (C) |
4 |
| (D) |
5 |
| 5- |
A $40 shirt now selling for $30 is discounted by what percent? |
| (A) |
25\% |
| (B) |
30\% |
| (C) |
35\% |
| (D) |
20\% |
| 6- |
Which of the following shows the numbers in descending order? |
| (A) |
\frac{3}{8}, \frac{1}{8}, \frac{2}{5}, \frac{1}{2} |
| (B) |
\frac{1}{8}, \frac{3}{8}, \frac{2}{5}, \frac{1}{2} |
| (C) |
\frac{1}{2}, \frac{3}{8}, \frac{2}{5}, \frac{1}{8} |
| (D) |
\frac{2}{5}, \frac{3}{8}, \frac{1}{8}, \frac{1}{2} |
| 7- |
The score of Emma was half as that of Ava and the score of Mia was twice that of Ava. If the score of Mia was 30, what is the score of Emma? |
| (A) |
5 |
| (B) |
15 |
| (C) |
10 |
| (D) |
20 |
| 8- |
If f=2 \ x \ - \ 6 \ y and g=x \ + \ 2 \ y, what is 2 \ f \ + \ g? |
| (A) |
5 \ x \ + \ 20 \ y |
| (B) |
2 \ x \ + \ 10 \ y |
| (C) |
5 \ x \ - \ 10 \ y |
| (D) |
- \ 5 \ x \ + \ 10 \ y |
| 9- |
712,232,691 \ × \ 0.001? |
| (A) |
71,223.2691 |
| (B) |
7,122,326.91 |
| (C) |
712,232.691 |
| (D) |
7,122.32691 |
| 10- |
one third of 12 is equal to \frac{1}{4} of what number? |
| (A) |
16 |
| (B) |
12 |
| (C) |
8 |
| (D) |
24 |
| 11- |
What is the mean in the following set of numbers?
7,15,26,32,48,63,100,121 |
| (A) |
51 |
| (B) |
51.5 |
| (C) |
54.5 |
| (D) |
54 |
| 12- |
Round off the result of 1.18 \ × \ 6.3 to the nearest tenth? |
| (A) |
7.40 |
| (B) |
7.43 |
| (C) |
7.44 |
| (D) |
7.41 |
| 13- |
Find \frac{1}{3} of \frac{5}{6} of 360? |
| (A) |
150 |
| (B) |
200 |
| (C) |
250 |
| (D) |
100 |
| 14- |
What is the value of x in the following equation?
3 \ (x \ + \ 5) = 2 \ (x \ − \ 1) \ + \ 15 |
| (A) |
- \ 2 |
| (B) |
2 |
| (C) |
5 |
| (D) |
3 |
| 15- |
What is the value of x in the following figure?
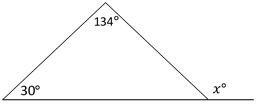 |
| (A) |
16^\circ |
| (B) |
132^\circ |
| (C) |
164^\circ |
| (D) |
150^\circ |
| 16- |
What is the value of x in the following figure?
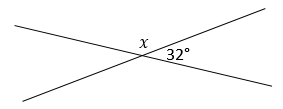 |
| (A) |
148^\circ |
| (B) |
152^\circ |
| (C) |
140^\circ |
| (D) |
125^\circ |
| 17- |
The ratio of boys and girls in a class is 2:5. If there are 49 students in the class, how many more boys should be enrolled to make the ratio 1:1? |
| (A) |
24 |
| (B) |
21 |
| (C) |
35 |
| (D) |
18 |
| 18- |
In five successive hours, a car travels 38 km, 40 km, 54 km, 32 km and 61 km. In the next five hours, it travels with an average speed of 54 km per hour. Find the total distance the car traveled in 10 hours. |
| (A) |
475 km |
| (B) |
425 km |
| (C) |
495 km |
| (D) |
455 km |
| 19- |
The price of a laptop is decreased by 12\% to $280. What is its original price? |
| (A) |
$200 |
| (B) |
$400 |
| (C) |
$300 |
| (D) |
$500 |
| 20- |
A company pays its employee $5,000 plus 5\% of all sales profit. If x is all sold profit, which of the following represents the employee’s revenue? |
| (A) |
0.5 \ x \ - \ 5,000 |
| (B) |
- \ 0.05 \ x \ - \ 5,000 |
| (C) |
0.05 \ x \ + \ 500 |
| (D) |
0.05 \ x \ + \ 5,000 |
| 21- |
The perimeter of the trapezoid below is 45. What is its area?
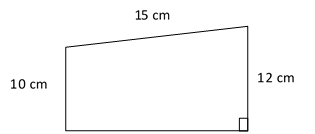 |
| (A) |
88 cm^2 |
| (B) |
82 cm^2 |
| (C) |
93 cm^2 |
| (D) |
79 cm^2 |
| 22- |
What is value of - \ 22 \ - \ (- \ 71)? |
| (A) |
29 |
| (B) |
39 |
| (C) |
49 |
| (D) |
59 |
| 23- |
Which of the following is a correct statement? |
| (A) |
\frac{3}{2} \ > \ 2.8 |
| (B) |
10\%=\frac{1}{5} |
| (C) |
4 \ < \ \frac{3}{6} |
| (D) |
\frac{2}{5} \ > \ 0.3 |
| 24- |
What is the area of a square whose diagonal is 6? |
| (A) |
36 |
| (B) |
30 |
| (C) |
32 |
| (D) |
38 |
| 25- |
Car A use 10-liter petrol per 100 kilometers; car B use 6-liter petrol per 100 kilometers. If both cars drive 350 kilometers, how much more petrol does car A use? |
| (A) |
28 |
| (B) |
14 |
| (C) |
24 |
| (D) |
12 |
| 26- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
x^2 \ + \ 15 \ =\ 64
124 \ - \ 15 \ y\ =\ 49
| Quantity A |
Quantity B |
| x |
y |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The two quantities are equal |
| (C) |
The quantity in Column A is greater |
| (D) |
The quantity in Column B is greater |
| 27- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
\frac{3}{4} \lt x \lt \frac{3}{2}
| Quantity A |
Quantity B |
| x |
\frac{4}{3} |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The two quantities are equal |
| 28- |
a and b are real numbers. a \lt b
| Quantity A |
Quantity B |
| |a \ - \ b| |
|b \ - \ a| |
|
| (A) |
The two quantities are equal |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The relationship cannot be determined from the information given |
| 29- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
| Quantity A |
Quantity B |
| \frac{x^5}{5} |
(\frac{x}{5})^5 |
|
| (A) |
The two quantities are equal |
| (B) |
The quantity in Column B is greater |
| (C) |
The quantity in Column A is greater |
| (D) |
The relationship cannot be determined from the information given |
| 30- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
| Column A |
Column B |
| 6 \ + \ 2 \times 7 \ + \ 5 |
4 \ + \ 4 \times 7 \ - \ 3 |
|
| (A) |
The quantity in Column B is greater |
| (B) |
The quantity in Column A is greater |
| (C) |
The two quantities are equal |
| (D) |
The relationship cannot be determined from the information given |
| 31- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
| Column A |
Column B |
| \sqrt{49} \ +\ \sqrt{64} |
\sqrt{81} |
|
| (A) |
The two quantities are equal |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The relationship cannot be determined from the information given |
| 32- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
| Column A |
Column B |
| \sqrt{121\ - \ 57} |
\sqrt{121}\ -\ \sqrt{57} |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The two quantities are equal |
| (C) |
The quantity in Column A is greater |
| (D) |
The quantity in Column B is greater |
| 33- |
The average age of Joe, Michelle, and Nicole is 24.
| Column A |
Column B |
| The average age of Joe and Michelle |
The average age of Michelle and Nicole |
|
| (A) |
The quantity in Column A is greater |
| (B) |
The quantity in Column B is greater |
| (C) |
The two quantities are equal |
| (D) |
The relationship cannot be determined from the information given |
| 34- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
\ y\ = \ - \ 5 \ x \ - \ 12
| Column A |
Column B |
| The value of x when y=8 |
- \ 9 |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The two quantities are equal |
| 35- |
A right cylinder with radius 4 inches has volume 36 \ π cubic inches.
| Quantity A |
Quantity B |
| The height of the cylinder |
4 inches |
|
| 36- |
The average of 5, 7, and x is 2.
| Quantity A |
Quantity B |
| x |
average of \ x\ ,\ x\ -\ 2 \ ,\ x\ + \ 6\ ,\ 4 \ x\ |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The two quantities are equal |
| 37- |
x is an integer greater than zero.
| Quantity A |
Quantity B |
| \frac{3}{x}\ + \ 2 \ x |
7 |
|
| (A) |
The quantity in Column A is greater |
| (B) |
The quantity in Column B is greater |
| (C) |
The two quantities are equal |
| (D) |
The relationship cannot be determined from the information given |
|
ISEE Middle Level
Practice Test 3
Mathematics Achievement
- 47 questions
- Total time for this section: 40 Minutes
- Calculators are not allowed at the test.
|
| 38- |
In a bundle of 60 pencils, 27 are red and the rest are blue. About what percent of the bundle is composed of blue pencils? |
| (A) |
55\% |
| (B) |
50\% |
| (C) |
60\% |
| (D) |
63\% |
| 39- |
Solving the equation: 10\ x\ -\ 12.5\ =- \ 42? |
| (A) |
-\ 2.95 |
| (B) |
-\ 3.18 |
| (C) |
-\ 2.54 |
| (D) |
3.43 |
| 40- |
What is the value of x in the following equation?
(x \ - \ 2)^3=125 |
| (A) |
5 |
| (B) |
7 |
| (C) |
- \ 2 |
| (D) |
- \ 5 |
| 41- |
The price of a car was $24,000 in 2014, \ $18,000 in 2015 and $12,500 in 2016. What is the rate of depreciation of the price of car per year? |
| (A) |
15\% |
| (B) |
20\% |
| (C) |
25\% |
| (D) |
30\% |
| 42- |
2 \ (\frac{1}{2} \ - \ \frac{1}{6})\ + \ 7? |
| (A) |
12 |
| (B) |
10 |
| (C) |
8 |
| (D) |
6 |
| 43- |
36 is equal to? |
| (A) |
2 \ + \ (3 \ × \ 10) \ + \ (2 \ × \ 30) |
| (B) |
(\frac{9}{3} \ × \ 2) \ + \ (\frac{30}{2} \ × \ 2) |
| (C) |
((\frac{3}{2} \ + \ 3) \ × \ \frac{18}{3}) \ + \ 63 |
| (D) |
(2 \ × \ 15) \ + \ (50 \ × \ 2) \ - \ 46 |
| 44- |
What number is 7 less than 40\% of 24? |
| (A) |
2.6 |
| (B) |
4.2 |
| (C) |
5.8 |
| (D) |
3.6 |
| 45- |
If a box contains red and blue balls in ratio of 3 : 5, how many red balls are there if 120 blue balls are in the box? |
| (A) |
59 |
| (B) |
64 |
| (C) |
81 |
| (D) |
72 |
| 46- |
If \frac{2 \ x}{5}=40, then \frac{2 \ x}{6} =? |
| (A) |
20 |
| (B) |
24 |
| (C) |
12 |
| (D) |
18 |
| 47- |
What is the difference in perimeter between a 6 cm by 5 cm rectangle and a circle with diameter of 12 cm? (π=3) |
| (A) |
16 cm |
| (B) |
12 cm |
| (C) |
10 cm |
| (D) |
24 cm |
$20.99 $15.99
24% Off*
A Comprehensive Review and Step-By-Step Guide to Preparing for the ISEE Middle Level Math Test
|
| 48- |
What number is 10 more than 12\% of 230? |
| (A) |
42 |
| (B) |
39.6 |
| (C) |
28.9 |
| (D) |
30 |
| 49- |
If 170\% of a number is 68, then what is the 80\% of that number? |
| (A) |
25 |
| (B) |
28 |
| (C) |
32 |
| (D) |
36 |
| 50- |
When a number is subtracted from 18 and the difference is divided by that number, the result is 3. What is the value of the number? |
| (A) |
3 |
| (B) |
5 |
| (C) |
6 |
| (D) |
9 |
| 51- |
Calculate the approximate area of the following circle.
 |
| (A) |
81.2 |
| (B) |
78.5 |
| (C) |
69.3 |
| (D) |
72.1 |
| 52- |
When a gas tank can hold 24 gallons, how many gallons does it contain when it is \frac{3}{4} full? |
| (A) |
24 |
| (B) |
20 |
| (C) |
18 |
| (D) |
15 |
| 53- |
From last year, the price of gasoline has increased from $1.25 per gallon to $1.75 per gallon. The new price is what percent of the original price? |
| (A) |
120\% |
| (B) |
160\% |
| (C) |
100\% |
| (D) |
140\% |
| 54- |
Which of the following angles can represent the three angles of an isosceles right triangle? |
| (A) |
45^\circ, 45^\circ, 90^\circ |
| (B) |
10^\circ, 80^\circ, 90^\circ |
| (C) |
50^\circ, 50^\circ, 80^\circ |
| (D) |
60^\circ, 60^\circ, 60^\circ |
| 55- |
If a gas tank can hold 42 gallons, how many gallons does it contain when it is \frac{5}{7} full? |
| (A) |
30 |
| (B) |
38 |
| (C) |
42 |
| (D) |
47 |
| 56- |
Which of the following is the greatest number? |
| (A) |
\frac{1}{2} |
| (B) |
\frac{5}{8} |
| (C) |
54\% |
| (D) |
\sqrt{0.36} |
| 57- |
A football team had $21,000 to spend on supplies. The team spent $12,000 on new balls. New sport shoes cost $120 each. Which of the following inequalities represent how many new shoes the team can purchase? |
| (A) |
120 \ x \ + \ 9,000 \ ≤ \ 21,000 |
| (B) |
120 \ x \ + \ 12,000 \ \geq \ 21,000 |
| (C) |
120 \ x \ + \ 12,000 \ ≤ \ 21,000 |
| (D) |
12,000 \ x \ + \ 120\ ≤ \ 21,000 |
| 58- |
In following rectangle which statement is true?
 |
| (A) |
Length of AB equal to length DC. |
| (B) |
AB is parallel to BC. |
| (C) |
The sum of all the angles equals 180^\circ. |
| (D) |
AB is perpendicular to DC. |
| 59- |
What is the missing term in the given numbers?
2, 3, 5, 8, 12, 17, ___, 30 |
| (A) |
36 |
| (B) |
30 |
| (C) |
25 |
| (D) |
23 |
| 60- |
The capacity of a red box is 18\% greater than a blue box. If the capacity of the red box is 59 books, how many books can be put in the blue box? |
| (A) |
60 |
| (B) |
68 |
| (C) |
58 |
| (D) |
50 |
| 61- |
What is the perimeter of a square that has an area of 49 square inches? |
| (A) |
36 inches |
| (B) |
28 inches |
| (C) |
14 inches |
| (D) |
30 inches |
| 62- |
[3 \times (–\ 18) \ +\ 6]\ – \ (– \ 8) \ + \ [2 \times 7] \div 2 = ? |
| (A) |
- \ 33 |
| (B) |
- \ 36 |
| (C) |
26 |
| (D) |
40 |
| 63- |
183 minutes = …? |
| (A) |
2.85 hours |
| (B) |
3.25 hours |
| (C) |
3.05 hours |
| (D) |
2.65 hours |
| 64- |
Two-kilograms apple and two-kilograms orange cost $27.3 If one-kilogram apple costs $3.2 how much does one-kilogram orange cost? |
| (A) |
$12.50 |
| (B) |
$15.25 |
| (C) |
$10.45 |
| (D) |
$8.45 |
| 65- |
Jason is 12 miles ahead of Joe running at 4.5 miles per hour and Joe is running at the speed of 6 miles per hour. How long does it take Joe to catch Jason? |
| (A) |
10 hours |
| (B) |
12 hours |
| (C) |
6 hours |
| (D) |
8 hours |
| 66- |
In a class, there are twice as many girls as boys. If the total number of students in the class is 69, how many girls are in the class? |
| (A) |
28 |
| (B) |
36 |
| (C) |
23 |
| (D) |
25 |
| 67- |
Jason left a $15.00 tip on a lunch that cost $50.00, approximately what percentage was the tip? |
| (A) |
30\% |
| (B) |
0.3\% |
| (C) |
27\% |
| (D) |
2.7\% |
| 68- |
At a Zoo, the ratio of lions to tigers is 2 to 5. Which of the following could NOT be the total number of lions and tigers in the zoo? |
| (A) |
56 |
| (B) |
72 |
| (C) |
99 |
| (D) |
96 |
| 69- |
Which of the following is not a prime number? |
| (A) |
103 |
| (B) |
101 |
| (C) |
97 |
| (D) |
58 |
| 70- |
(((- \ 15) \ +\ 24)\ ×\ 2)\ +\ (- \ 21)? |
| (A) |
- \ 1 |
| (B) |
2 |
| (C) |
- \ 3 |
| (D) |
3 |
| 71- |
If 50\% of a class are girls, and 36\% of girls play tennis, what percent of the class play tennis? |
| (A) |
18\% |
| (B) |
12\% |
| (C) |
24\% |
| (D) |
33\% |
| 72- |
A shaft rotates 200 times in 5 seconds. How many times does it rotate in15 seconds? |
| (A) |
600 |
| (B) |
400 |
| (C) |
540 |
| (D) |
620 |
| 73- |
The price of a sofa is decreased by 30\% to $420. What was its original price? |
| (A) |
$600 |
| (B) |
$560 |
| (C) |
$500 |
| (D) |
$480 |
| 74- |
The width of a rectangle is 2 \ x and its length is 5 \ x. The perimeter of the rectangle is 42. What is the value of x? |
| (A) |
3 |
| (B) |
5 |
| (C) |
7 |
| (D) |
9 |
| 75- |
What is the area of the trapezoid?
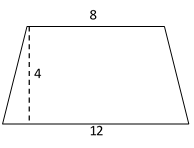 |
| (A) |
50 |
| (B) |
40 |
| (C) |
30 |
| (D) |
20 |
| 76- |
Ella bought a pair of gloves for $12.49. She gave the clerk $18.00. How much change should she get back? |
| (A) |
$5.51 |
| (B) |
$6.23 |
| (C) |
$8.78 |
| (D) |
$4.98 |
| 77- |
\frac{7\times12}{80} is closest estimate to: |
| (A) |
1.1 |
| (B) |
6.1 |
| (C) |
3.4 |
| (D) |
2.9 |
| 78- |
\frac{3}{4}\ +\ \frac{\frac{-\ 2}{5}} {\frac{4}{10}}=? |
| 79- |
What is the value of in the following equation?
10\ +\ 4\ (\ x\ +\ 5\ -\ 5\ x\ )=30 |
| (A) |
0 |
| (B) |
2 |
| (C) |
8 |
| (D) |
5 |
| 80- |
A swimming pool holds 2,000 cubic feet of water. The swimming pool is 25 feet long and 10 feet wide. How deep is the swimming pool? |
| (A) |
8 feet |
| (B) |
4 feet |
| (C) |
9 feet |
| (D) |
3 feet |
| 81- |
If 60\% of A is 20\% of B, then B is what percent of A? |
| (A) |
300\% |
| (B) |
200\% |
| (C) |
250\% |
| (D) |
350\% |
| 82- |
12.124\div0.002= ? |
| (A) |
6,062 |
| (B) |
6,132 |
| (C) |
9,278 |
| (D) |
7,342 |
| 83- |
A card is drawn at random from a standard 52–card deck, what is the probability that the card is of Hearts? (The deck includes 13 of each suit clubs, diamonds, hearts, and spades) |
| (A) |
\frac{ 1}{4} |
| (B) |
\frac{ 1}{3} |
| (C) |
\frac{2}{3} |
| (D) |
\frac{5}{6} |
| 84- |
Solve the following equation?
6^x=1,296 |
| (A) |
4 |
| (B) |
10 |
| (C) |
12 |
| (D) |
7 |