|
ISEE Middle Level
Practice Test 4
Quantitative Reasoning
- 37 questions
- Total time for this section: 35 Minutes
- Calculators are not allowed at the test.
|
| 1- |
If an object travels at \(0.2\) cm per second, how many meters does it travel in \(5\) hours? |
| (A) |
\(35.8\) m |
| (B) |
\(36\) m |
| (C) |
\(40\) m |
| (D) |
\(38\) m |
| 2- |
Ava uses a \(28\%\) off coupon when buying a sweater that costs \($42.21\). If she also pays \(4\%\) sales tax on the purchase, how much does she pay? |
| (A) |
\($30.42\) |
| (B) |
\($28.35\) |
| (C) |
\($36.20\) |
| (D) |
\($31.60\) |
| 3- |
In the following shape \(y\) equals to?
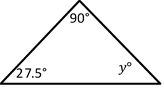 |
| (A) |
\(52.3^°\) |
| (B) |
\(62.5^°\) |
| (C) |
\(45.2^°\) |
| (D) |
\(48.6^°\) |
| 4- |
What’s the circumference of a circle that has a diameter of \(12\) m? |
| (A) |
\(30.14\) m |
| (B) |
\(35\) m |
| (C) |
\(37.68\) m |
| (D) |
\(42.20\) m |
| 5- |
Find \(\frac{1}{2}\) of \(\frac{ 2}{5}\) of \(\frac {4}{7}\) of \(210\)? |
| (A) |
\(48\) |
| (B) |
\(32\) |
| (C) |
\(28\) |
| (D) |
\(24\) |
| 6- |
If the ratio of home fans to visiting fans in a crowd is \(3:2\) and all \(25,000\) seats in a stadium are filled, how many visiting fans are in attendance? |
| (A) |
\(5,000\) |
| (B) |
\(8,000\) |
| (C) |
\(10,000\) |
| (D) |
\(15,000\) |
| 7- |
Which of the following shows the numbers in increasing order? |
| (A) |
\(\frac{3}{7},\frac{5}{7},\frac{8}{9},\frac{3}{2}\) |
| (B) |
\(\frac{5}{7},\frac{3}{7},\frac{8}{9},\frac{3}{2}\) |
| (C) |
\(\frac{3}{2},\frac{5}{7},\frac{8}{9},\frac{3}{7}\) |
| (D) |
\(\frac{8}{9},\frac{3}{2},\frac{3}{7},\frac{5}{7}\) |
| 8- |
What is the value of \(x\) in the following equation?
\(\frac{5^x}{125}=3,125\) |
| (A) |
\(8\) |
| (B) |
\(9\) |
| (C) |
\(5\) |
| (D) |
\(3\) |
| 9- |
An item in the store originally priced at \($250\) was marked down \(15\%\). What is the final sale price of the item? |
| (A) |
\($212.5\) |
| (B) |
\($220\) |
| (C) |
\($210.3\) |
| (D) |
\($200\) |
| 10- |
A shirt costing \($230\) is discounted \(20\%\). After a month, the shirt is discounted another \(20\%\). Which of the following expressions can be used to find the selling price of the shirt |
| (A) |
\((230)\ (0.20) \ (0.20)\) |
| (B) |
\((184)\ (0.85) \ (0.85)\) |
| (C) |
\((230)\ (0.80) \ (0.80)\) |
| (D) |
\((184)\ (0.80) \ (0.80)\) |
| 11- |
If \(x\leq a\) is the solution of \(6 \ +\ 3 \ x \leq 12\), what is the value of \(a\)? |
| (A) |
\(2\) |
| (B) |
\(4\) |
| (C) |
\(x\) |
| (D) |
\(2 \ x\) |
| 12- |
What is the area of the shaded region? (one forth of the circle is shaded)
Diameter = \(18\)
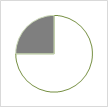 |
| (A) |
\(24.25 \ π\) |
| (B) |
\(20.25 \ π\) |
| (C) |
\(22.50 \ π\) |
| (D) |
\(25.50 \ π\) |
| 13- |
\(3\) liters of water are poured into an aquarium that's \(15\) cm long, \(5\) cm wide, and \(70\) cm high. How many cm will the water level in the aquarium rise due to this added water? (\(1\) liter of water \(= 1000\)cm\(^3\)) |
| (A) |
\(30\) cm |
| (B) |
\(25\) cm |
| (C) |
\(54\) cm |
| (D) |
\(40\) cm |
| 14- |
What is the perimeter of the following parallelogram?
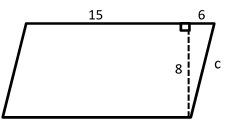 |
| (A) |
\(58\) |
| (B) |
\(39\) |
| (C) |
\(74\) |
| (D) |
\(62\) |
| 15- |
The area of the trapezoid below is \(108\). What is the value of \(x\)?
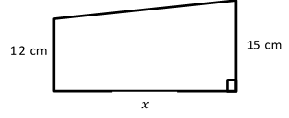 |
| (A) |
\(8\) |
| (B) |
\(11\) |
| (C) |
\(6\) |
| (D) |
\(16\) |
| 16- |
If a car has \(50-\)liter petrol and after one hour driving the car use \(4-\)liter petrol, how much petrol remaining after \(x-\)hours? |
| (A) |
\(50\ - \ \frac{4}{2} \ x\) |
| (B) |
\(50\ - \ 4 \ x\) |
| (C) |
\(50\ + \ 4 \ x\) |
| (D) |
\(50\ + \ x\) |
| 17- |
What is the value of \(\frac{\frac{-\ 10}{2} \ \times \ \frac{3}{5}}{\frac{12}{21}}\) = ? |
| (A) |
\(- \ \frac{40}{231}\) |
| (B) |
\(- \ \frac{231}{40}\) |
| (C) |
\(- \ \frac{231}{30}\) |
| (D) |
\(- \ \frac{221}{30}\) |
| 18- |
If Joe was making \($4.50\) per hour and got a raise to \($4.75\) per hour, what percentage increase was the raise? |
| (A) |
\(3.33\%\) |
| (B) |
\(4.44\%\) |
| (C) |
\(5.55\%\) |
| (D) |
\(7.77\%\) |
| 19- |
Solve for \(x: \ 4 \ +\ x\ +\ 12 \ (\frac{x}{4})=6 \ x\ +\ 15\) |
| (A) |
\(- \ \frac{7}{2}\) |
| (B) |
\(\frac{13}{2}\) |
| (C) |
\(- \ \frac{ 11}{2}\) |
| (D) |
\(- \ 5\) |
| 20- |
What is the value of mode and median in the following set of numbers?
\(\ 1\ ,\ 2\ ,\ 2\ ,\ 5\ ,\ 4\ ,\ 4\ ,\ 3\ ,\ 3\ ,\ 3\ ,\ 1\ ,\ 1\) |
| (A) |
Mode:\(1, 2\) Median:\(2\) |
| (B) |
Mode:\(2, 3\) Median:\(2\) |
| (C) |
Mode:\(1, 3\) Median:\(3\) |
| (D) |
Mode:\(1, 3\) Median:\(2, 5\) |
| 21- |
The average of \(\ 12\ , \ 16\ , \ 24\) and \( \ x\) is \( 13\). What is the value of \(x\)? |
| (A) |
\(0\) |
| (B) |
\(- \ 2\) |
| (C) |
\(2\) |
| (D) |
\(1\) |
| 22- |
Which is the equivalent temperature of \(104^°\)F in Celsius? (C \(=\) Celsius)
\(C = \frac{3}{5} \ (F \ – \ 54)\) |
| (A) |
\(40^\circ\) |
| (B) |
\(45^\circ\) |
| (C) |
\(35^\circ\) |
| (D) |
\(30^\circ\) |
| 23- |
\(6\) less than five times a positive integer is \(29\). What is the integer? |
| (A) |
\(7\) |
| (B) |
\(5\) |
| (C) |
\(3\) |
| (D) |
\(- \ 3\) |
| 24- |
In a bundle of \(25\) fruits, \(12\) are apples and the rest are bananas. What percent of the bundle is composed of apples? |
| (A) |
\(48\%\) |
| (B) |
\(25\%\) |
| (C) |
\(54\%\) |
| (D) |
\(39\%\) |
| 25- |
If \( 2 \ f\ +\ 4\ g\ =6 \ x\ + \ 2 \ y\) and \(\ g\ =2\ y\ -\ 5 \ x\), what is \(f\)? |
| (A) |
\(10 \ x\ + \ 2 \ y\) |
| (B) |
\(13\ x\ -\ 2 \ y\) |
| (C) |
\(13\ x\ + \ 3 \ y\) |
| (D) |
\(\frac{8}{13} \ x\ - \ 2 \ y\) |
| 26- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
| Column A |
Column B |
| \(4^3\ -\ 3^4 \) |
\(3^4\ -\ 4^3\) |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The two quantities are equal |
| 27- |
Using the information provided below, compare the quantity in column A to the quantity in Column B. A
| Column A |
Column B |
| The slope of the line \(\ 3\ x\ +\ 5\ y\ =\ 16\) |
The slope of the line that passes through points \((1, 5)\) and \((2, 3)\) |
|
| (A) |
The two quantities are equal |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The relationship cannot be determined from the information given |
| 28- |
Using the information provided below, compare the quantity in column A to the quantity in Column B. .
| Column A |
Column B |
| \(\sqrt{225\ -\ 81}\) |
\(\sqrt{225}\ -\ \sqrt{81}\) |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The two quantities are equal |
| (C) |
The quantity in Column A is greater |
| (D) |
The quantity in Column B is greater |
| 29- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
\(3 \ x^5\ - \ 7=425\)
\(\frac{1}{3}\ -\ \frac{y}{5}=-\ \frac{8}{15}\)
| Column A |
Column B |
| \(x \) |
\(y\) |
|
| (A) |
The quantity in Column A is greater |
| (B) |
The quantity in Column B is greater |
| (C) |
The two quantities are equal |
| (D) |
The relationship cannot be determined from the information given |
| 30- |
Using the information provided below, compare the quantity in column A to the quantity in Column B. \(x\) is a positive number..
| Quantity A |
Quantity B |
| \(x^{15}\) |
\(x^{30}\) |
|
| (A) |
The quantity in Column A is greater |
| (B) |
The quantity in Column B is greater |
| (C) |
The two quantities are equal |
| (D) |
The relationship cannot be determined from the information given |
| 31- |
Using the information provided below, compare the quantity in column A to the quantity in Column B. \(12\) percent of \(x\) is equal to \(15\) percent of \(y\), where \(x\) and \(y\) are positive numbers.
| Quantity A |
Quantity B |
| \(x\) |
\(y\) |
|
| (A) |
The quantity in Column A is greater |
| (B) |
The quantity in Column B is greater |
| (C) |
The two quantities are equal |
| (D) |
The relationship cannot be determined from the information given |
| 32- |
Using the information provided below, compare the quantity in column A to the quantity in Column B. A computer costs \($300\).
| Column A |
Column B |
| A sales tax at \(10\%\) of the computer cost |
\($30\) |
|
| (A) |
The two quantities are equal |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The relationship cannot be determined from the information given |
| 33- |
Using the information provided below, compare the quantity in column A to the quantity in Column B..
| Quantity A |
Quantity B |
| \((-\ 6^3)\) |
\(6^3\) |
|
| (A) |
The two quantities are equal |
| (B) |
The relationship cannot be determined from the information given |
| (C) |
The quantity in Column A is greater |
| (D) |
The quantity in Column B is greater |
| 34- |
Using the information provided below, compare the quantity in column A to the quantity in Column B..
| Quantity A |
Quantity B |
| \((1.23)^5 \ (1.23)^4\) |
\((1.23)^{9}\) |
|
| (A) |
The quantity in Column A is greater |
| (B) |
The quantity in Column B is greater |
| (C) |
The two quantities are equal |
| (D) |
The relationship cannot be determined from the information given |
| 35- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.
| Column A |
Column B |
| \(\frac{\sqrt{64\ +\ 36}}{\sqrt{25\ -\ 9}}\) |
\(\frac{8\ -\ 3}{9\ -\ 2}\) |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The two quantities are equal |
| 36- |
Using the information provided below, compare the quantity in column A to the quantity in Column B..
| Quantity A |
Quantity B |
| The least prime factor of \(55\) |
The least prime factor of \(330\) |
|
| (A) |
The relationship cannot be determined from the information given |
| (B) |
The quantity in Column A is greater |
| (C) |
The quantity in Column B is greater |
| (D) |
The two quantities are equal |
| 37- |
Using the information provided below, compare the quantity in column A to the quantity in Column B.The sum of \(2\) consecutive integers is \(- \ 24\) .
| Column A |
Column B |
| The largest of these integers |
\(-\ 12\) |
|
| (A) |
The quantity in Column A is greater |
| (B) |
The quantity in Column B is greater |
| (C) |
The two quantities are equal |
| (D) |
The relationship cannot be determined from the information given |
|
ISEE Middle Level
Practice Test 4
Mathematics Achievement
- 47 questions
- Total time for this section: 40 Minutes
- Calculators are not allowed at the test.
|
| 38- |
Which of the following is not synonym for \(10^2\)? |
| (A) |
the square of \(10\) |
| (B) |
\(10\) to the second power |
| (C) |
\(10\) cubed |
| (D) |
\(10\) squared |
| 39- |
If the area of trapezoid is \(169\), what is the perimeter of the trapezoid?
 |
| (A) |
\(49\) |
| (B) |
\(35\) |
| (C) |
\(52\) |
| (D) |
\(40\) |
| 40- |
If \(x=\) lowest common multiple of \(42\) and \(66\) then \(\frac{x}{2}\ +\ 1\)equal to? |
| (A) |
\(217\) |
| (B) |
\(307\) |
| (C) |
\(285\) |
| (D) |
\(232\) |
| 41- |
Which of the following angles is obtuse? |
| (A) |
\(86^\circ\) |
| (B) |
\(130^\circ\) |
| (C) |
\(215^\circ\) |
| (D) |
\(74^\circ\) |
| 42- |
Anita’s trick–or–treat bag contains \(12\) pieces of chocolate, \(18\) suckers, \(18\) pieces of gum,\( 24\) pieces of licorice. If she randomly pulls a piece of candy from her bag, what is the probability of her pulling out a piece of sucker |
| (A) |
\(\frac{1}{4}\) |
| (B) |
\(\frac{2}{5}\) |
| (C) |
\(\frac{3}{8}\) |
| (D) |
\(\frac{1}{2}\) |
| 43- |
In two successive years, the population of a town is increased by \(15\%\) and \(20\%\) . What percent of the population is increased after two years? |
| (A) |
\(44\%\) |
| (B) |
\(32\%\) |
| (C) |
\(38\%\) |
| (D) |
\(40\%\) |
$20.99 $15.99
24% Off*
A Comprehensive Review and Step-By-Step Guide to Preparing for the ISEE Middle Level Math Test
|
| 44- |
If angles A and B are angles of a parallelogram, what is the sum of the measures of the two angles? |
| (A) |
Cannot be determined |
| (B) |
\(360\) degrees |
| (C) |
\(180\) degrees |
| (D) |
\(90\) degrees |
| 45- |
What is the value of \(x\) in the following equation?
\(4^{x \ - \ 1} \ -\ 38=218\) |
| (A) |
\(3\) |
| (B) |
\(2\) |
| (C) |
\(5\) |
| (D) |
\(7\) |
| 46- |
\(12 \ +\ 4 \times (–\ 4) \ –\ [2 \ +\ 25 \times 3] \div 11 = ?\) |
| (A) |
\( 11\) |
| (B) |
\(-\ 13\) |
| (C) |
\(-\ 11\) |
| (D) |
\(13\) |
| 47- |
Solve the following equation?
\((\ x^2\ +\ 2\ x\ +\ 1\ )=64\) |
| (A) |
\(7,- \ 11\) |
| (B) |
\(7,- \ 9\) |
| (C) |
\(9,- \ 11\) |
| (D) |
\(- \ 9,11\) |
| 48- |
\(\frac{12 \ × \ 24}{7}\) is closest estimate to? |
| (A) |
\(39.2\) |
| (B) |
\(41.1\) |
| (C) |
\(41.8\) |
| (D) |
\(39.8\) |
| 49- |
A swing moves from one extreme point (point A) to the opposite extreme point (point B) in \(28\) seconds. How long does it take that the swing moves \(10\) times from point A to point B and returns to point A? |
| (A) |
\(500\) seconds |
| (B) |
\(560\) seconds |
| (C) |
\(600\) seconds |
| (D) |
\(640\) seconds |
| 50- |
When a number is multiplied to itself and added by \(12\), the result is \(76\). What is the value of the number? |
| (A) |
\(-\ 5 \) and \(5\) |
| (B) |
\(-\ 6 \) and \(6\) |
| (C) |
\(-\ 8 \) and \(8\) |
| (D) |
\(8\) |
| 51- |
Solving the equation: \(\frac{x}{3}\ +\ \frac{11}{3}=\frac{13}{6}=\)? |
| (A) |
\(- \ 4\ \frac{1}{2}\) |
| (B) |
\(- \ 4 \ \frac{3}{2}\) |
| (C) |
\(- \ 4 \ \frac{9}{4}\) |
| (D) |
\(- \ 4\ \sqrt{\frac{1}{2}}\) |
| 52- |
In \(1999\) , the average worker's income increased \($3,000\) per year starting from \($22,000\) annual salary. Which equation represents income greater than average?
(\( I =\) income, \(x =\) number of years after \(1999\)) |
| (A) |
\(I \gt 3000 \ x\ +\ 22000\) |
| (B) |
\(I \gt 3000 \ x\ - \ 20000\) |
| (C) |
\(I \gt 2000 \ x\ + \ 20000\) |
| (D) |
\(I \leq 3000 \ x\ + \ 22000\) |
| 53- |
What is ratio of perimeter of figure \(A\) to area of figure \(B\)?
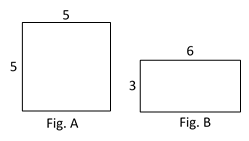 |
| (A) |
\(\frac{5}{9}\) |
| (B) |
\(\frac{12}{18}\) |
| (C) |
\(\frac{10}{9}\) |
| (D) |
\(\frac{25}{18}\) |
| 54- |
There are \(2\) cars moving in the same direction on a road. A red car is \(12\) km ahead of a blue car. If the speed of the red car is \(60\) km per hour and the speed of the blue car is \(1\ \frac{3}{4}\) of the red car, how many minutes will it take the blue car to catch the red car? |
| (A) |
\(60\) minutes |
| (B) |
\(90\) minutes |
| (C) |
\(45\) minutes |
| (D) |
\(30\) minutes |
| 55- |
What is the absolute value of the quantity five minus nine? |
| (A) |
\(- \ 4\) |
| (B) |
\(- \ 2\) |
| (C) |
\(2\) |
| (D) |
\(4\) |
| 56- |
Which of the following angles can represent the three angles of an equilateral triangle? |
| (A) |
\(50^°, 50^°, 80^°\) |
| (B) |
\(45^°, 45^°, 90^°\) |
| (C) |
\(60^°, 60^°, 60^°\) |
| (D) |
\(10^°, 80^°, 90^°\) |
| 57- |
\(125 \ \frac{1}{2}\) is equal to? |
| (A) |
\((\frac{28}{ 4} \ +\ \frac{8}{2} ) \times 9 \ −\ \frac{9}{ 2} \ +\ \frac{124}{ 4}\) |
| (B) |
\(20 \ −\ (4 \ ×\ 10) \ +\ (6 \times 30)\) |
| (C) |
\((\frac{11} {8} \times 72)\ +\ (\frac{125}{ 5} )\) |
| (D) |
\((2 \times 10) \ +\ (50 \times 1.5) \ +\ 15\) |
| 58- |
What is the difference in area between a \(12\) cm by \(4\) cm rectangle and a circle with diameter of \(12\) cm ? \((π=3)\) |
| (A) |
\(66\) |
| (B) |
\(42\) |
| (C) |
\(18\) |
| (D) |
\(60\) |
| 59- |
Mr. Jones saves \($4,500\) out of his monthly family income of \($40,000\). What fractional part of his income does he save? |
| (A) |
\(\frac{9}{80}\) |
| (B) |
\(\frac{1}{80}\) |
| (C) |
\(\frac{1}{20}\) |
| (D) |
\(\frac{9}{20}\) |
| 60- |
If you invest \($8,000\) at an annual rate of \(11\%\), how much interest will you earn after one year? |
| (A) |
\($880\) |
| (B) |
\($88\) |
| (C) |
\($80\) |
| (D) |
\($800\) |
| 61- |
Which is not a prime number? |
| (A) |
\(151\) |
| (B) |
\(131\) |
| (C) |
\(121\) |
| (D) |
\(181\) |
| 62- |
There are three boxes, a red box, a blue box, and a yellow box. If the weight of the red box is \(80\) kg and the weight of the red box is \(90\%\) of the weight of the blue box, and the weight of the blue box is \(120\%\) of the weight of the yellow box, what is the weight of blue and yellow boxes respectively? |
| (A) |
\(100\) and \(80\) |
| (B) |
\(110\) and \(70\) |
| (C) |
\(80\) and \(100\) |
| (D) |
\(70\) and \(70\) |
| 63- |
Each of the students in a team may invite up to \(6\) friends to a party. What is the maximum number of students and guests who might attend the party? |
| (A) |
\(5\ x\) |
| (B) |
\(6\ x\) |
| (C) |
\(7\ x\) |
| (D) |
\(8\ x\) |
| 64- |
How many possible outfit combinations come from five shirts, three slacks, and ten ties? |
| (A) |
\(100\) |
| (B) |
\(150\) |
| (C) |
\(250\) |
| (D) |
\(300\) |
| 65- |
If \(y = 3\ a\ b \ + \ 2\ b^3\), what is y when \(a = 2\) and \(b = 4\)? |
| (A) |
\(216\) |
| (B) |
\(327\) |
| (C) |
\(211\) |
| (D) |
\(189\) |
| 66- |
In the figure below, line A is parallel to line B. What is the value of angle \(x\)?
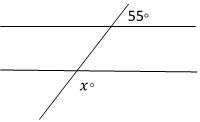 |
| (A) |
\(135\) degree |
| (B) |
\(145\) degree |
| (C) |
\(115\) degree |
| (D) |
\(125\) degree |
| 67- |
Three people go to a restaurant. Their bill comes to \($94.00\). They decided to split the cost. One person pays \($17.5\), the next person pays \(3\) times that amount. How much will the third person have to pay? |
| (A) |
\($30\) |
| (B) |
\($17.5\) |
| (C) |
\($22\) |
| (D) |
\($24\) |
| 68- |
\(210\) minutes=…? |
| (A) |
\(4.5\) Hours |
| (B) |
\(3.5\) Hours |
| (C) |
\(5\) Hours |
| (D) |
\(3\) Hours |
| 69- |
Two-kilogram apple and three-kilograms orange cost \($35\) If price of one-kilogram of apple is twice price of one-kilogram of orange. How much does one kilogram apple cost? |
| (A) |
\($8\) |
| (B) |
\($5\) |
| (C) |
\($10\) |
| (D) |
\($15\) |
| 70- |
In the following equation, what is the value of \(3 \ x\ +\ 2\ y\)?
\(x\ +\ 2\ x\ -\ 15=2\ (\frac{9}{2} \ x\ +\ 2 \ y)\ -\ 12\) |
| (A) |
\(- \ \frac{3}{2 }\) |
| (B) |
\(- \ \frac{1}{2 }\) |
| (C) |
\(\frac{1}{2 }\) |
| (D) |
\(2\) |
| 71- |
What is the perimeter of the below right triangle?
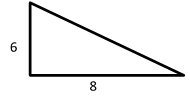 |
| (A) |
\(30\) |
| (B) |
\(36\) |
| (C) |
\(24\) |
| (D) |
\(21\) |
| 72- |
The average weight of \(18\) girls in a class is \(60\) kg and the average weight of \(32\) boys in the same class is \(62\) kg. What is the average weight of all the \(50\) students in that class? |
| (A) |
\(60.76\) |
| (B) |
\(80.10\) |
| (C) |
\(61.28\) |
| (D) |
\(73.48\) |
| 73- |
How many tiles of \(9\) cm\(^2\) is needed to cover a floor of dimension \(12\) cm by \(18\) cm? |
| (A) |
\(18\) |
| (B) |
\(12\) |
| (C) |
\(21\) |
| (D) |
\(24\) |
| 74- |
What is in the following right triangle?
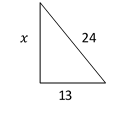 |
| (A) |
\(\sqrt{403}\) |
| (B) |
\(\sqrt{401}\) |
| (C) |
\(20\) |
| (D) |
\(\sqrt{407}\) |
| 75- |
Calculate the approximate circumference of the following circle.
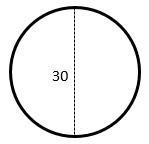 |
| (A) |
\(94\) |
| (B) |
\(76\) |
| (C) |
\(83\) |
| (D) |
\(91\) |
| 76- |
\((((-\ 16)\ +\ 36)\ ×\ \frac{1}{4})\ +\ (-\ 12)\)? |
| (A) |
\(-\ 5\) |
| (B) |
\(-\ 7\) |
| (C) |
\(8\) |
| (D) |
\(\frac{3}{5}\) |
| 77- |
What is the value of \((16 \ - \ 12)!\)? |
| (A) |
\(24\) |
| (B) |
\(18\) |
| (C) |
\(30\) |
| (D) |
\(39\) |
| 78- |
John traveled \(120\) km in \(4\) hours and Alice traveled \(180\) km in \(5\) hours. What is the ratio of the average speed of John to average speed of Alice? |
| (A) |
\(5 : 9\) |
| (B) |
\(9 : 5\) |
| (C) |
\(6 : 5\) |
| (D) |
\(5 : 6\) |
| 79- |
What is the difference of smallest \(4\)–digit number and biggest \(4\)–digit number? |
| (A) |
\(8974\) |
| (B) |
\(6774\) |
| (C) |
\(8999\) |
| (D) |
\(6989\) |
| 80- |
You just drove \(510\) miles and it took you approximately \(12\) hours. How many miles per hour was your average speed? |
| (A) |
\(42.5\) miles per hour |
| (B) |
\(45.5\) miles per hour |
| (C) |
\(44\) miles per hour |
| (D) |
\(41.5\) miles per hour |
| 81- |
An angle is equal to one seventh of its supplement. What is the measure of that angle? |
| (A) |
\(22.5^\circ\) |
| (B) |
\(24^\circ\) |
| (C) |
\(34^\circ\) |
| (D) |
\(34.5^\circ\) |
| 82- |
If \(140\%\) of a number is equal to \(30\%\) of \(90\) , then what is the number? |
| (A) |
\(19.28\) |
| (B) |
\(16.22\) |
| (C) |
\(19.12\) |
| (D) |
\(18.52\) |
| 83- |
In a department of a company, the ratio of employees with Bachelor’s degree to employees with high school Diploma is \(2\) to \(5\). If there are \(18\) employees with Bachelor’s degree in this department, how many employees with High School Diploma should be moved to other departments to change the ratio of the number of employees with Bachelor’s Degree to the number of employees with High School Diploma to \(3\) to \(4\) in this department? |
| (A) |
\(39\) |
| (B) |
\(21\) |
| (C) |
\(28\) |
| (D) |
\(54\) |
| 84- |
What is \(41,8410\) in scientific notation? |
| (A) |
\(4.1841\times10^5\) |
| (B) |
\(4.1841\times10^3\) |
| (C) |
\(4184.1\times10^4\) |
| (D) |
\(41.841\times10^6\) |